
Applied Dynamics in Engineering
1 469 kr
1 469 kr
Ons, 18 jun - ons, 25 jun
Säker betalning
14-dagars öppet köp
Säljs och levereras av
AdlibrisProduktbeskrivning
Features
· The analysis of the structure of the differential equation of motion, as well as the analysis of the components that constitute this equation presented in the Chapter 1 allow readers to understand the principles of composing the differential equation of motion for actual engineering systems.
· Presents the straightforward universal methodology of solving linear differential equations of motion based on the Laplace transform.
· The table of Laplace Transform pairs presented in the Chapter 1 is based on reviewing numerous related analytical sources and represents a comprehensive source containing sufficient information for solving the differential equations of motion for common engineering systems.
· Helps determine the number of possible common engineering problems based on the analysis of the structure of the differential equation of motion, as well as on the realistic resisting and active loading factors that constitute the differential equation of motion.
· Each paragraph represents a standalone description. There is no need to look for notations or analytical techniques throughout the book. The book contains all required supplemental information for solving the problems. In his two-book, combined presentation of dynamics, Applied Dynamics in Engineering (2016) and Solving Engineering Problems in Dynamics (2014), Dr. Michael Spektor sets up, for anyone interested in the subject, a unique approach, facilitating an intuitive understanding of dynamics in application to design.
Rather than a traditional vector approach to the topic, he presents a linear systems treatment. There are many advantages of this approach, especially as an introductory course in dynamic system analysis and design and particularly in an engineering technology curriculum where a student has only one semester’s exposure to the subject. Of advantage to students is how Spektor progresses from the most fundamental dynamic system configurations of inertial mass, spring compliance, and friction to those of wide application in machinery.
With Dr. Spektor’s presentation of dynamical concepts, the design implications are always front and center. The student proceeds through fully documented and extraordinarily detailed examples of every applicable system. All mathematical detail is related to the Laplace Transform solution of linear differential equations, which has universal application in measurement, instrumentation, and electric circuitry. Unavoidable mathematical complexities also are covered in the shorter companion volume. For engineering technology students, this approach to learning dynamics directly builds on and parallels the formal mathematical training they are applying in other analytical subjects.
I would have loved it if this book had been available when I was first learning dynamics, and I look forward adopting it in an Engineering Technology curriculum.—Carl Wolf, Project Manager, Small Step Innovation, LLC The book Applied Dynamics in Engineering by Michael Spektor, presents the solutions for engineering problems in a variety of applied topics. The range of material presented illustrates the in-depth background of the author.
The focus of the book is the use of differential equations as a foundation for mathematical/engineering solutions. The author talks in the language of a teacher; his phrases are exact in nature and most pleasant to read.
The use of Laplace Transformation is well illustrated, and the inclusion of a table for Laplace Transform Pairs is very useful. The solution for partial differential equations is well served by this text as well. In fact, the author’s use of this solution technique is most impressive.
In summary, this is an excellent teaching or reference book for the student or professional engineer.—Wallace Shakun, Former Dean of Technology, Clayton State University, Georgia
Modern technology is rapidly changing, requiring the application of the most effective methods of improvement and development of engineering systems. These methods comprise the purposeful analytical investigations of mechanical and related systems in the area of applied dynamics. The main role of analytical investigations in the area of dynamics related to engineering systems consists of providing the possibility for purposeful control of the parameters of the systems in order to obtain the required performance of the system during the executing of the working process. Michael Spektor’s Applied Dynamics in Engineering addresses these issues.—Professor Walter Buchanan, Texas A&M University
In 54 years as a mechanical engineer and scholar, I have never had Laplace Transforms laid out for me in a more complete and understandable manner than it is by Michael Spektor in his two-volume set of books Solving Engineering Problems in Dynamics and Applied Dynamics in Engineering.
Way back in the final year of my doctoral studies, I was advised that I ought to have a graduate-level math course listed among my studies. I found a course that was scheduled to be taught the next semester entitled something like “Transform Calculus.” I had A grades in calculus, and I had been a “whiz kid” with the Fourier series, so I signed up for it.
There was a lot on my mind that semester. I found the course to be abstract and diverting. My disinterest was duly rewarded with a C grade—the only C that I had received since my freshman year. Since it was the only course that I took that term, I was surprised to receive a letter advising me that I was on academic probation and would have to take another graduate course the next semester and get an A grade to average out that C. I chose another highly technical course and got the A.
Though professionally, I subsequently used other transform methods both analytical and experimental, the incident left me terrified of the Laplace Transform. My fear continued to stalk me, even though shortly afterward in my career I successfully held the title of “Structural Dynamics Engineer” with a Fortune 500 company.
So I was again surprised when Dr. Michael Spektor, my long-time friend and colleague for 26 years, told me that over his 10 years of retirement, he had just completed the two above-cited books devoted to the use of Laplace Transforms in the solution of mechanical engineering and technology problems. I knew Michael to be a very accurate and successful professor and department head. And I was familiar with his research work on designing a vibration machine to penetrate soil. But Dr. Spektor’s new and independent scholarship on the use of the Laplace Transform is profound.
He has searched the literature on transforms that would be specific to the study and practice of mechanical engineering only. And he reduces his findings to 96 transform pairs that meet the specific needs of mechanical engineers. This I learned from him as I now enter the final year before my own retirement from teaching. I expect that checking through some of his many transform pairs will be an early pleasure of my own retirement and my own overdue conquest of this, my personal Chimera.—Professor Lawrence J. Wolf, Oregon Institute of Technology
Artikel.nr.
5ac77ebe-d619-440b-a980-735bd97b355b
Applied Dynamics in Engineering
1 469 kr
1 469 kr
Ons, 18 jun - ons, 25 jun
Säker betalning
14-dagars öppet köp
Säljs och levereras av
AdlibrisLiknande toppsäljare

POP MART Labubu The Monsters – Exciting Macaron Blind Box 17 cm Vinylfigur | Samlarobjekt | Designer Toy | Originalprodukt
179 kr

1 st POP MART Labubu The Monsters Exciting Macaron Plyschfigur Leksak Blind Box (slumpad färg, 17 cm, 1 pack)
179 kr

Samsung Galaxy Buds3 Pro - Silver
1 640 kr

POP MART Labubu The Monsters Big into Energy 17 cm
199 kr

1 st POP MART Labubu 2.0 The Monsters Macaron Blind Box Plyschfigur (slumpad färg, 17 cm, generation 2, 1-pack)
199 kr
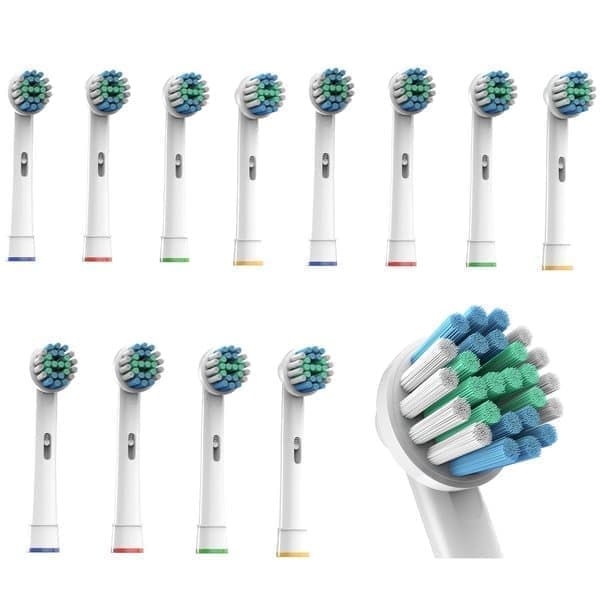
12-pack Oral-B Kompatibla Tandborsthuvuden
89 kr

Sony PlayStation DualSense - White (PS5)
639 kr
Tidigare lägsta pris:
679 kr

Trådlös CarPlay Adapter 2025, för CarPlay och Android Auto, Plug-and-Play
225 kr

Elastiskt träningsband till fotboll - Gul/Svart
114 kr
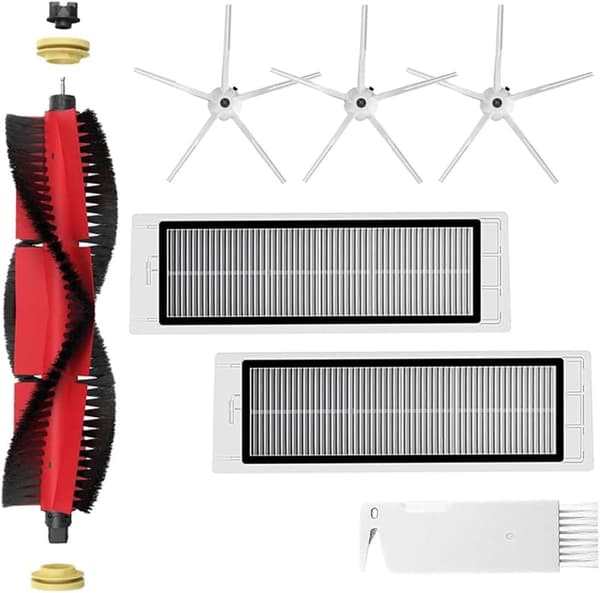
INF Tillbehör för Roborock S5/S6 modeller 7 delar
149 kr
Tidigare lägsta pris:
199 kr
Rekommendationer för dig

Playstation 5 DualSense Controller Midnight Black
639 kr
Tidigare lägsta pris:
679 kr

INF TYPE-C Dubbel SD/TF-kortläsare för snabb dataöverföring 0
79 kr

Apple AirPods 4 Active Noise Cancellation Wireless In-ear
2 096 kr
Tidigare lägsta pris:
2 099 kr

UNIQ XL Hollywood Spegel med 15 LED-lampor och touch-funktion - sminkspegel med belysning - hollywoodspegel
795 kr
Tidigare lägsta pris:
895 kr

Samsung Galaxy Tab A9+ Wifi 64GB Svart grafit
1 980 kr

iPhone Snabbladdare USB-C PD 3.0. 20W Strömadapter + Kabel
117 kr

Apple Iphone 16e 128GB Svart Svart
7 390 kr

Vattenfontän solcellsdriven 16 cm solcellsfontän vatten fontän utomhus Svart
149 kr
Tidigare lägsta pris:
179 kr

Laddare för iPhone 15 / iPhone 16 + 2M kabel Snabbladdare USB-C till USB-C
99 kr

INF Cocktail set Dubbel shaker 750ml Rostfritt stål Silver 10 delar
289 kr
Tidigare lägsta pris:
385 kr